最高のコレクション Sqrt(x^2+y^2) Graph 265099-Square Root Of X^2 + Y^2 Graph

Math Input Use Math Input Mode to directly enter textbook math notation Try it

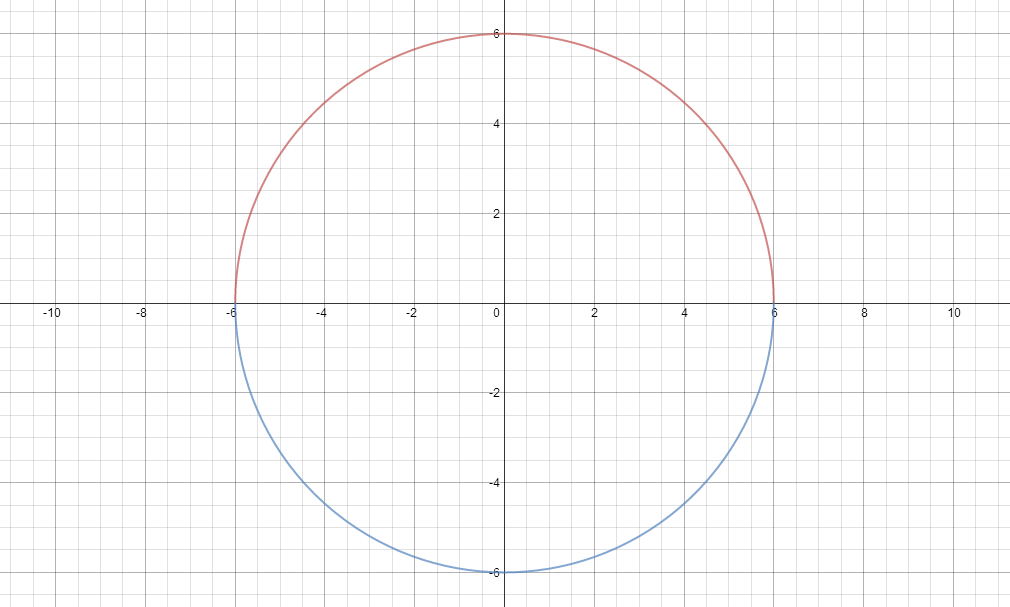
Square root of x^2 + y^2 graph-To graph the XY plane you set Z = 0 and plot the function as you normally would, so $$z = \sqrt(x^2 y^2 1) == 0 = \sqrt(x^2 y^2 1)$$ $$\text {Therefore} x^2 y^2 = 1$$ is your XY axis graph, which is just a circle of radius 1 centered at the origin Hello, Let Sigma the surface of your function F it's a surface of revolution because F(x,y) = f(r) where r = sqrt(x^2y^2) Precisely, f(r) = sqrt(r^21) ln(4r^2) First, plot the curve of f r \mapsto sqrt(r^2 1) ln(4r^2) You get Now, turn this curve around zaxes in 3Dspace You get the surface Sigma

Square root of x^2 + y^2 graphのギャラリー

各画像をクリックすると、ダウンロードまたは拡大表示できます

ページ番号をクリックして他の画像を表示し、画像をクリックして画像のダウンロードリンクを取得します

「Square root of x^2 + y^2 graph」の画像ギャラリー、詳細は各画像をクリックしてください。

ページ番号をクリックして他の画像を表示し、画像をクリックして画像のダウンロードリンクを取得します

「Square root of x^2 + y^2 graph」の画像ギャラリー、詳細は各画像をクリックしてください。

ページ番号をクリックして他の画像を表示し、画像をクリックして画像のダウンロードリンクを取得します

「Square root of x^2 + y^2 graph」の画像ギャラリー、詳細は各画像をクリックしてください。

ページ番号をクリックして他の画像を表示し、画像をクリックして画像のダウンロードリンクを取得します

「Square root of x^2 + y^2 graph」の画像ギャラリー、詳細は各画像をクリックしてください。

ページ番号をクリックして他の画像を表示し、画像をクリックして画像のダウンロードリンクを取得します

「Square root of x^2 + y^2 graph」の画像ギャラリー、詳細は各画像をクリックしてください。

ページ番号をクリックして他の画像を表示し、画像をクリックして画像のダウンロードリンクを取得します

「Square root of x^2 + y^2 graph」の画像ギャラリー、詳細は各画像をクリックしてください。

ページ番号をクリックして他の画像を表示し、画像をクリックして画像のダウンロードリンクを取得します

「Square root of x^2 + y^2 graph」の画像ギャラリー、詳細は各画像をクリックしてください。

ページ番号をクリックして他の画像を表示し、画像をクリックして画像のダウンロードリンクを取得します

「Square root of x^2 + y^2 graph」の画像ギャラリー、詳細は各画像をクリックしてください。

 As for your request in the comments, one can write the function as sqrt(u*v), where u=xy and v=xy Then x=(uv)/2 and y=(uv)/2 Since the variables are just placeholders in a parametric plot, we can rename u and v to x and y, respectively, and arrive atYou could use y = x2 with explicit domain 0,∞) as the parent graph graph { (sqrt (x)/sqrt (x))x^2 4767, 523, 06, 44} Reflecting this in the diagonal line y = x we get the graph of y = √x graph {sqrt (x) 4767, 523, 06, 44} George C 1

Incoming Term: sqrt(x^2+y^2) graph, root x^2+y^2 graph, z=sqrt(x^2+y^2) graph, f(x y)=sqrt(x^2+y^2) graph, square root of x^2 + y^2 graph, graph of cone z=sqrt(x^2+y^2), graph z=sqrt(1-x^2-y^2), graph z=sqrt(4-x^2-y^2), 3d graph 1.2+(sqrt(1 sqrt(x^2+y^2 2))), x^2+(y-3 sqrt(x^2))^2=1 graph,